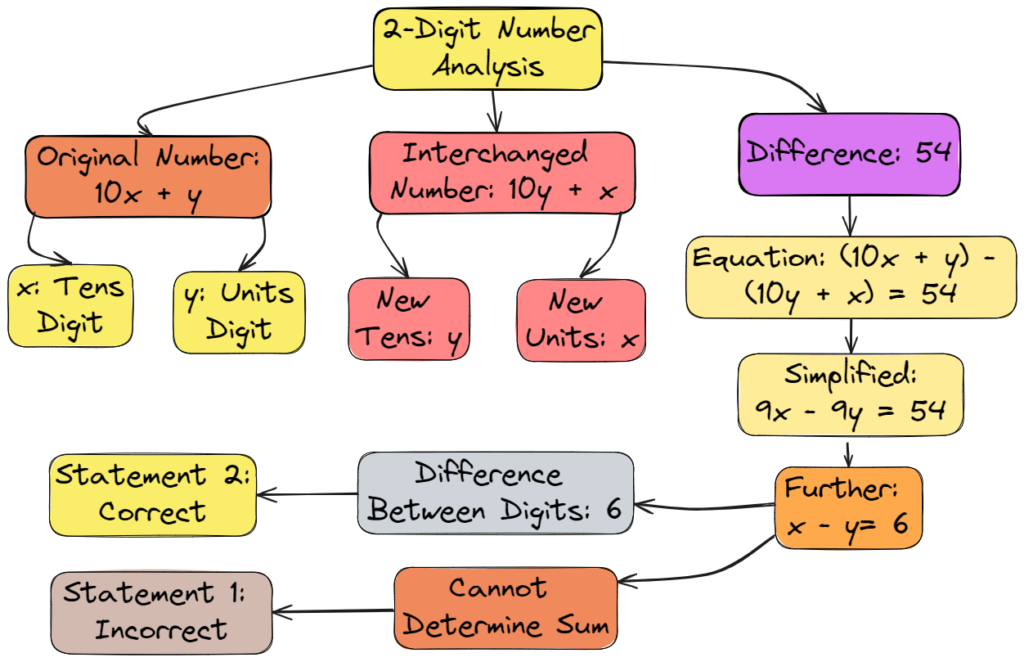
Q. The difference between a 2-digit number and the number obtained by interchanging the positions of the digits is 54.
1. The sum of the two digits of a number can be determined only if the product of the two digits is known.
2. The difference between the two digits of the number can be determined.
Which of the above statements is/are correct?
(a) 1 only
(b) 2 only
(c) Both 1 and 2
(d) Neither 1 nor 2
Correct Answer: (b) 2 only
Question from UPSC Prelims 2021 CSAT Paper
Explanation :
The difference between 2 digit number and the number obtained is 54
Given:
– Original number = 10x + y (where x is tens digit, y is units digit)
– New number after interchanging = 10y + x
– Difference between these numbers = 54
Step 1: Write equation for the difference
(10x + y) – (10y + x) = 54
Step 2: Simplify left side
10x + y – 10y – x = 54
9x – 9y = 54
Step 3: Further simplify
9(x – y) = 54
x – y = 6
Step 4: Analyze what we know
– Difference between tens and units digit is 6
– Since it’s a 2-digit number:
* x must be from 1 to 9
* y must be from 0 to 9
* x must be greater than y by 6
Step 5: Find possible digit pairs
Since x – y = 6:
– If y = 0, x = 6
– If y = 1, x = 7
– If y = 2, x = 8
– If y = 3, x = 9
Analysis:
1. Statement 1 (about sum of digits) is incorrect
– Multiple pairs of digits satisfy x – y = 6
– Each pair would give different sums
– Examples: 6+0=6, 7+1=8, 8+2=10, 9+3=12
2. Statement 2 (about difference of digits) is correct
– We proved x – y = 6
– This is a definite value
Answer: Statement 1 is incorrect and Statement 2 is correct.